The optimum theory attempts to define what would economically be the ideal size of population for a particular country. According to the optimum theory, there is a particular size of population, which along with the existing natural resources and a given state of technology yields the highest income per capita in a country.
- a) Under population: If population of a country is below the optimum size, the country is said to be under-populated. In under populated country, the natural and capital resources are not fully exploited (utilized).
- b) Over population: If the population is in excess of optimum size, the country is said to be over populated. Following are some of the problems to be faced by over-populated country.
Average productivity will decrease.
Per capita income will be very low.
Standard of living will fall.
If the quantity of labour is small relative to the natural resources, then even the actually available resources remain under-utilized. If the population increases and more labourers become available to be combined with the given stock of the natural resources and capital equipments, out put per capita or per capita income will rise. As population continues to increase, a point will finally be reached when capital and natural resources are fully utilized and, therefore, output per capita is the highest. The level of population at which per capita output (income) is the maximum is called the optimum population. If population still goes on increasing, that is, crosses the optimum point, out put per capita will start declining. The country would then become over-populated.
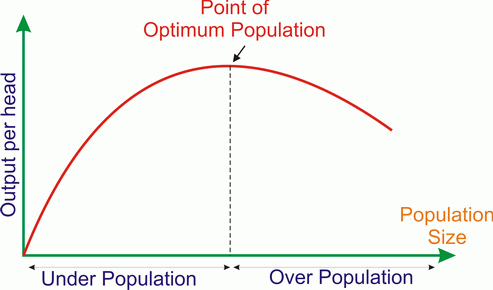
Fig a: Optimum population
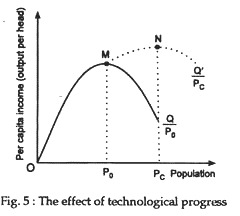
Fig b: Shifts in optimum population
In the figure a, at OM level of population, the output per capita (MP) is the highest. If the population increases beyond OM, output per capita falls. Therefore, OM is the optimum population. If the population of the country is less than OM, it will be under-populated and if the population is more than OM, it will be a case of over population. The per capita out put curve may change (M’P’ and M” P”) as a result of an increase in resources or progress in technology and their effects on optimum population are shown in the figure b. That is, the size of optimum population also increases. Dalton has given a formula with which we can judge the extent to which the actual population of a country deviates from the optimum population. The extent of deviation is called mal-adjustment. The formula is M = A-O / O. Where M is maladjustment, A is actual population and O is optimum population. If M is negative, the country is under-populated. If M is zero, the country has optimum population and if M is positive, the country is over populated. For instance, if the actual population is 80 crores and the optimum population is 40 crores, then M=80-40 / 40 =1. This indicates that the country is over populated.
Criticisms of Optimum theory of population
a) It is almost impossible to determine the optimum size of population, as it is very difficult to estimate the level of capital stock available in a country.
b) As the natural and capital resources continuously change, the size of optimum population is also subject to change.
